Fig. 5.1
Sound waves (longitudinal wave) propagate through compression and rarefactions of the medium
With respect to ultrasound, the wavelength is an extremely important parameter as the wavelength affects both the type of reflection that occurs as well as the axial resolution of the image. As depicted in Fig. 5.2a, b, within the same medium, higher frequencies result in shorter wavelengths while lower frequencies result in longer wavelengths.
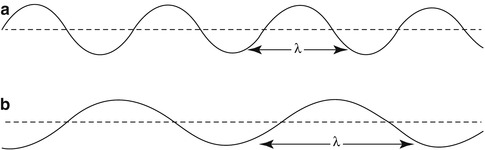

Fig. 5.2
(a) Higher frequency and shorter wavelength. (b) Lower frequency and longer wavelength
Acoustic Power and Intensity
The power of the wave relates to the pressure developed within the tissue. In order to produce the sound waves, a piezoelectric transducer is excited by an electrical signal, referred to as the transmit voltage. Higher transmit voltages produce higher acoustic pressure fields, increasing the strength of the reflected signal as well as increasing the maximum depth of penetration. Of course, because of the physical interaction with the medium, a higher acoustic pressure potentially increases the risk of causing tissue damage (referred to as a bioeffect). The parameter that is measured to assess both the ability to improve signal strength and the risk of inducing a bioeffect is the intensity. The intensity is a measure of the distribution of the power per unit area, as indicated by Eq. 5.1. Higher intensities increase sensitivity and penetration but also increase the risk of causing thermal bioeffect (an increase in temperature that can cause protein denaturing leading to cell death) and mechanical bioeffects referred to as cavitation (a rapid phase transition which creates cavities that can implode within the tissue).
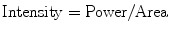
(5.1)
Propagation Velocity and Roundtrip Time
Another important wave parameter is the propagation velocity. The propagation velocity is the speed at which a wave travels through a medium and is determined by the properties of the medium. In essence, more dense tissues tend to be stiffer (higher bulk modulus) which results in higher propagation velocities. Ultrasound machines start with a simplified assumption that sound travels at 1,540 m/s (1.54 mm/μs). Based on this assumption and simple application of the distance equation, the time for sound to travel 1 cm in the body is calculated to be approximately 6.5 μs. Since ultrasound imaging is based on signal reflection, the roundtrip effect must be taken into account, which implies that 13 μs are required for each 1 cm of imaging depth (2 cm roundtrip travel) (Fig. 5.3). Of course, when the actual speed of sound varies significantly enough from the assumed 1,540 m/s, error exists with the displayed image (portraying structures too shallow when higher than the assumed speed and too deep when slower than the presumed speed).
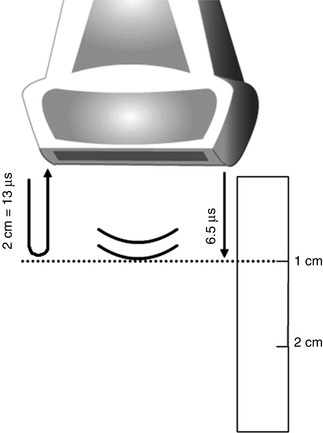

Fig. 5.3
Roundtrip effect and imaging travel time per centimeter (Reprinted from “Essentials of Ultrasound Physics: the Board Review Book,” by F. R. Miele, p. 327. Copyright 2008 by Miele Enterprises, LLC. Reprinted with permission)
Reflection
Reflection from tissues is dependent on geometric and acoustic properties. Geometrically, when the reflecting surface is large and smooth with respect to the wavelength, a very angle-dependent type of reflection, specular reflection, occurs. When the surface is rough relative to the wavelength, the much less angle-sensitive (back) scattering occurs. Finally, when the reflecting structures are small relative to the wavelength (as occurs with red blood cells), Rayleigh scattering occurs. Note that reflection from a needle is highly specular and hence highly angle dependent. For specular reflection, the ideal angle is an incident angle of 0° (which implies that the beam direction is perpendicular to the reflecting structure) (Fig. 5.4).
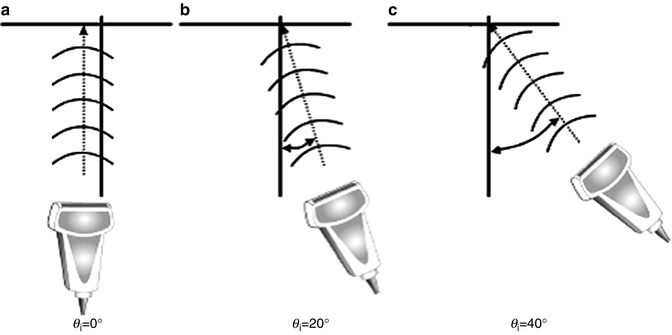

Fig. 5.4
Incident angle (Reprinted from “Ultrasound Physics & Instrumentation,” by F.R. Miele, p. 147. Copyright 2006 by Miele Enterprises, LLC. Reprinted with permission)
As the angle increases (the beam direction relative to the reflective surface becomes more acute), an increasing percentage of the reflected energy is lost, decreasing the ability to visualize the reflecting structure. Eventually, at large enough incident angles, the reflecting structure will not be at all visualized. This change in visualization as a result of angle-dependent reflection is referred to as anisotropy. Practically speaking, this fact has ramifications when dealing with ultrasound-guided needle procedures. The ideal incident angle of 0° is generally not possible. By using a shallower entrance approach, the incident angle remains smaller and the needle is generally better visualized. The incident angle can be somewhat manipulated by rocking the transducer (what is sometimes referred to as heel-to-toe rocking) and/or by changing the electronic B-mode steering (for those systems that offer this capability). Note that the ability to adequately visualize tendons and nerves is also very angle dependent.
Percentage Reflection and Acoustic Properties
The percentage of the sound wave energy that reflects at a boundary between two media is determined by the disparity between the acoustic properties of the adjoining tissues (Eq. 5.2). This property is referred to as the acoustic impedance. The acoustic impedance of a medium is directly proportional to the density and the propagation velocity of the medium. At a boundary between two mediums, when a large mismatch (difference) in acoustic impedances exists, the percentage of the sound energy reflected is high. Of course, as more energy reflects from a particular point, there is less energy to insonify the inferior tissues (lower transmission). When the impedance mismatch is smaller, less reflection (and hence more transmission) occurs. It is important to note that higher impedance mediums do not imply high reflection percentages. As specified in the equation, the amount of reflection is related to the difference in impedances such that transitioning from a high to a low impedance, or from a low to a high impedance, results in large reflections. When the sound wave transitions from a high impedance medium to another high impedance medium, very little reflection occurs. Similarly, when the sound wave propagates from a medium with a low acoustic impedance to another medium with a low impedance, very little reflection occurs. This fact explains why fresh thrombus comprised primarily of red blood cells of the same acoustic properties may not be visualized whereas older clot, especially when calcified, generally presents with a very echogenic appearance.
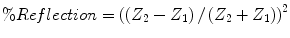
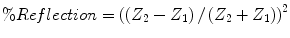
(5.2)
Application of the reflection equation is useful in predicting and understanding the acoustic appearance of various tissue structures. Relative to the acoustic impedance of muscle, the acoustic impedance of tendon and bone is quite high, resulting in very strong reflection (hyperechoic images). In comparison, fat has a lower acoustic impedance than muscle and therefore tends to produce lower acoustic echoes. Acoustically, blood appears relatively “homogenous” such that the blood pool itself typically produces very little reflection (hypoechoic). Note that when fluid-filled cystic structure is insonified, the boundary of the fluid represents a very large acoustic impedance mismatch and hence produces a strong echo; however, when the fluid itself is homogenous, no reflection occurs, and hence, no internal echoes are visualized (anechoic). At the other extreme from fluids, metallic structures such as needles and surgical clips represent very high impedances. Relative to the lower impedance of the surrounding tissue, the high impedance of these metallic objects results in very large reflection percentages and, hence, very strong bright echoes.
For ultrasound-guided needle procedures, visualization of nerves is obviously very important. Sonographically, nerves commonly have a honeycomb appearance in cross section, which translates to a striated appearance longitudinally. The explanation for this appearance, of course, is explained by the reflection equation (Eq. 5.2) in conjunction with the properties of nerves. A nerve consists of a bundle of fascicles. The bundle is encased with the epineurium, and each fascicle is encased by perineural connective tissue. The nerve fascicles generally appear hypoechoic surrounded by more hyperechoic perineural connective tissue. The epineurium is also echogenic.
5.2.2 Transducers, Absorption, and Resolution
Ultrasound transducers are created from piezoelectric materials. Piezoelectric materials convert mechanical stress (such as occurs with mechanical vibration from sound waves) into electrical energy (voltage). These materials also exhibit “reciprocity” which means that the same crystal can convert electrical energy into mechanical energy. Transducers operate over a range of frequencies, referred to as the transducer bandwidth as depicted in Fig. 5.5. This bandwidth allows for imaging at different frequencies without swapping transducers as well as allowing for different operating frequencies for different ultrasound modalities (i.e., imaging at 5 MHz while performing Doppler at 3.0 MHz). High-frequency ultrasound generally has the advantage of superior axial (depth), lateral (side by side), and elevation (slice thickness) resolution. The disadvantage of high-frequency imaging is significantly less penetration as a result of increased absorption (the conversion of sound energy into heat within the tissue). Absorption rates increase exponentially with increasing transmit frequency and increasing depth, implying that using even slightly higher frequencies can significantly decrease penetration and signal strength at deeper imaging depths. Broadband transducers offer the flexibility without having to change out transducers of transmitting with lower frequency for improved sensitivity and penetration at greater depths as well as transmitting at higher frequencies for improved resolution for shallower imaging.


Fig. 5.5
Bandwidth (Reprinted from “Essentials of Ultrasound Physics: the Board Review Book,” by F.R. Miele, p. 327. Copyright 2008 by Miele Enterprises, LLC. Reprinted with permission)
Transducers can be constructed from single crystals (referred to as pencil probes, pedofs, or Doppler only) or multiple elements to form phased arrays. Phased array transducers consist of different form factors including:
Sectors, which bring the image to a point designed for rib access and used generally in cardiac imaging although also used for transcranial and, sometimes, abdominal imaging
Curved linears, which produce broad near field by a curved surface, generally used on the abdomen and for invasive transducers such as transrectal and transvaginal
Linears, which have a large flat surface ideal for contact with relatively flat surfaces such as the neck, arms, and legs. Linear transducers have three different imaging formats including unsteered, steered, and trapezoidal (Fig. 5.6)

Fig. 5.6
Linear transducer imaging formats (Reprinted from “Ultrasound Physics & Instrumentation,” by F.R. Miele, pp. 277–279. Copyright 2006 by Miele Enterprises, LLC. Reprinted with permission)
5.2.3 Linear Phased Arrays
Since venous imaging is performed primarily with linear phased arrays, we will emphasize the functionality of linear arrays. Linear images are produced by activating a group of elements, referred to as the aperture, to produce a single beam. Generally the same aperture is then used to receive over time the returning echoes. Based on the depth setting, the system determines the required receive time, referred to as the pulse repetition period (PRP) before transmitting the next line. Recall that 13 μs is required for each cm of imaging depth such that the PRP is simply the imaging depth multiplied by 13 μs/cm. Once the required “listen” time has transpired, the system activates another group of elements to produce the next acoustic beam and the process is repeated until the user-specified region of the patient is scanned. The frequency at which the lines can be transmitted, the pulse repetition frequency (PRF) is equal to the reciprocal of the pulse repetition period (PRP). Once the entire region is scanned, the system goes back to the beginning and repeats the process, creating the next frame of data. The time required to acoustically produce a frame is simply calculated as the time required to produce a line (the PRP) multiplied by the number of lines that constitute the frame. The reciprocal of the frame time is the frame frequency, commonly referred to as the frame rate. The frame rate is one of the predominant determinants of the ability to resolve changes in time, or the temporal resolution. Higher frame rates imply better temporal resolution. Higher frame rates can be achieved by minimizing the time required to produce the frame. Clearly narrowing the image and decreasing the depth setting decrease the frame time and improve temporal resolution.
5.3 Ultrasound Imaging
5.3.1 Operating Frequency
Changing the transmitted wave parameters can have significant impact on the image quality and clinical value of the data acquired. As already mentioned, most modern systems offer broad bandwidth transducers that allow the user to select the operating frequency. Typically, the frequency range for linear transducers is between about 2.5 MHz and about 15 MHz. This range is too broad for any one transducer and is generally covered by either two or three different transducers (i.e., a specific vendor may offer broadband linear transducers with frequency ranges of 2.5–7, 5–12, and 8–15 MHz). For imaging, the general rule for operating frequency selection is to choose the highest frequency that still results in adequate penetration. Following this rule ensures that resolution is optimized while still presenting adequate signal above noise thresholds. Practically speaking, there can only be general and not specific rules about appropriate frequency selection for conventional imaging, since attenuation with depth varies from patient to patient.
5.3.2 Transmit Power
For deeper imaging, as occurs with deeper vessels on large patients, the transmit power should be increased. For the superficial venous system, the transmit power can generally be reduced from the maximum without compromise of image quality.
5.3.3 Transmit Focus
A third important system setting which affects sensitivity is the transmit focus or foci. When imaging superficially with only one focus, the focus should be set at the depth of vein or artery being assessed. For deep veins when inadequate sensitivity is a potential issue, the focus should be set just slightly deeper than the vein of interest since, for deeper imaging depths, the highest beam intensity is generally shallower than the focal depth. Using multiple foci improves the lateral resolution and sensitivity over the focal range and in general is very useful for venous imaging. The downside of using multiple foci is that image acquisition slows, decreasing the frame rate, hence, degrading temporal resolution.
5.3.4 Signal and Gain
The visualization of grayscale signals on the screen is the result of a complex interaction between multiple systems and environmental settings including the receiver gain, time gain compensation (TGC), compression settings, monitor contrast and brightness, and the ambient lighting of the room. Before discussing the approach to setting the system controls, it is imperative to first discuss the ultrasound signal relative to the light perception of the human eye. First, there is a need for signal amplification since even the highest amplitude signals reflecting from the body are too small to be adequately visualized. The system control, known as “receiver gain,” is basically a multiplier that increases the amplitude of all received signals. TGC is also receiver gain but differs in how the gain is applied. Unlike the receiver gain that multiplies all signals, TGC is depth dependent. Signals that are received from deeper depths arrive later in time and generally require more amplification than signals from shallower depths. TGC allows for the image to be subdivided into zones based on depth, and then different amplification applied to each of these zones to compensate for the varying attenuation.
5.3.5 Signal and Compression
Reflected ultrasound signals span a very large range of intensities (referred to as the “signal dynamic range”). Reflections from mediums such as nerves, tendons, bones, and calcifications tend to be of very high amplitude as a result of the large acoustic impedance mismatches relative to the surrounding tissues. Conversely, reflections from within blood, fluids, and fresh thrombus tend to be of low amplitude as a result of the relative acoustic impedance homogeneity. The dynamic range of these reflected signals often spans 80 or even 100 dB (a factor in amplitude of 10,000:1 and 100,000:1). The human eye for a given ambient light is capable of detecting less than 36 dB (fewer than 64 shades of gray). The result is that the dynamic range of reflected signals from diagnostic ultrasound far exceeds the dynamic range of the human eye. This fact illustrates why compression must be employed in displaying ultrasound images. As the name suggests, compression reduces the dynamic range by mathematically remapping the wider range of signal amplitudes to a smaller range of signal amplitudes. The problem is, that by compressing the dynamic range of reflected signals, it is more than conceivable that some information may be lost and an important differentiation between tissue types may be missed (i.e., an inability to differentiate a mass from surrounding normal tissue or an inability to visualize a thrombus from the surrounding blood pool).
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree


