Mitral valve (MV) repair has become the preferred treatment for mitral regurgitation associated with degenerative MV disease. Although the functional benefits of early MV repair are known, the associated alterations in left ventricular (LV) shape have not been studied. The aim of this study was to evaluate serial changes in LV performance and 3-dimensional (3D) shape after MV repair using a new analytic technique. Fifty patients (mean age 59 ± 12 years) with severe asymptomatic mitral regurgitation and ejection fractions >55% who underwent MV repair were enrolled. Transthoracic real-time 3D echocardiography was performed the day before and 6 and 12 months after MV repair. An age-matched control group of 50 normal subjects was studied for comparison. Endocardial surfaces were extracted to compute 3D shape indexes of sphericity and conicity, which were compared in patients between different time points and versus the normal group. Postoperatively, LV volumes were decreased compared to presurgical values. Ejection fractions were slightly decreased 6 months after surgery but had restored by 12 months. These changes were associated with modifications in end-diastolic LV shape: before surgery, compared to normal controls, sphericity was augmented and conicity was decreased; at 6 months, these shape changes were reversed, with no further improvements at 12 months. In conclusion, patients with asymptomatic mitral regurgitation and preserved LV function already exhibit changes in LV shape. Early MV repair leads to near normal morphology after surgery, indicating the benefits of this procedure. Real-time 3D echocardiography and novel 3D shape analysis allow detailed serial examination of the complex relation between LV performance and shape.
It has been shown that real-time 3-dimensional (3D) echocardiography allows the rapid acquisition of 3D data sets, thereby providing the basis for accurate volumetric measurements of left ventricular (LV) size, function, and mass. However, although currently available commercial software allows reliable measurements of 3D LV volumes, no tools are available for the quantitative evaluation of 3D LV shape. To fill this gap, we recently proposed a new technique to quantify LV morphology by the computation of 3D shape indexes from LV endocardial surfaces extracted from real-time 3D echocardiographic data sets. We hypothesized that this method could be applied to describe the serial subtle changes that occur in LV morphology after mitral valve (MV) repair, thereby providing additional insights into postsurgical LV remodeling. Accordingly, the aims of this study were (1) to assess changes in LV shape immediately after MV repair and during 1-year follow-up in asymptomatic patients with severe mitral regurgitation due to degenerative MV prolapse and (2) to compare the results to those obtained in a group of normal subjects.
Methods
We studied 100 subjects, divided into 2 groups: (1) 50 patients (36 men, mean age 59 ± 12 years) with degenerative MV disease and severe mitral regurgitation, enrolled at Centro Cardiologico Monzino (Milan, Italy) who underwent surgical MV repair, and (2) 50 age-matched normal subjects (37 men, mean age 57 ± 9 years) enrolled at the University of Chicago Hospitals. Mitral regurgitation was defined as severe in the presence of effective regurgitant orifice area ≥0.4 cm 2 , estimated using the proximal isovelocity surface area technique, according to recent recommendations. Clinical characteristics of the study groups are listed in Table 1 . The protocol was approved by the institutional review boards of the 2 institutions, and informed consent was obtained from all study subjects.
| Variable | Controls (n = 50) | MV Repair (n = 50) |
|---|---|---|
| Age (years) | 57 ± 9 | 59 ± 12 |
| Men/women | 37/13 | 36/14 |
| Body surface area (m 2 ) | 1.7 ± 0.2 | 1.8 ± 0.2 |
| Ring size (mm) | — | 30 ± 2 |
| Medications | — | 50 (100%) |
| β blockers | 11 (22%) | |
| Angiotensin-converting enzyme inhibitors | 15 (30%) | |
| Diuretics | 41 (82%) | |
| Acetylsalicylic acid | 8 (16%) | |
| Statins | 6 (12%) | |
| Amiodarone | 20 (40%) | |
| Ca 2+ antagonists | 5 (10%) | |
| Angiotensin receptor blocker | 5 (10%) |
Exclusion criteria were the presence of (1) atrial fibrillation and cardiac arrhythmias, (2) an ejection fraction <55% (biplane Simpson’s rule from 2-dimensional transthoracic echocardiographic data), (3) associated MV stenosis, or (4) a history of endocarditis, (5) coronary artery disease (significant coronary artery stenosis, previous myocardial infarction, bypass graft surgery, or coronary stent implantation). All patients underwent coronary angiography before surgery to exclude asymptomatic coronary artery disease. Additional exclusion criteria for the normal subjects were (1) abnormal global and regional LV function, (2) cardiac medications, (3) a history of systemic hypertension or coronary artery disease, and (4) the presence of valvular heart disease (mitral regurgitation more than mild).
All patients underwent complete 2-dimensional (S5 probe; Philips Medical Systems, Andover, Massachusetts) and 3D transthoracic echocardiographic examinations the day before and at 6 and 12 months after MV repair. Immediately before surgery, complete intraoperative 2-dimensional transesophageal echocardiographic examinations were performed (model 21354A or iE33; Philips Medical Systems) to assess the morphology of the MV apparatus. After repair, intraoperative transesophageal echocardiography was performed to assess for residual mitral regurgitation, mitral stenosis, and systolic anterior motion of the MV. The technique of MV repair varied according to morphologic MV findings and the preference of the 2 surgeons involved in the study. In all cases, ring annuloplasty was performed to stabilize the annulus and the suture line. MV repair was considered successful only when the grade of residual mitral regurgitation was less than or equal to mild. In case of postrepair MV regurgitation greater than mild, the patient was excluded from the study.
Normal subjects underwent single 2-dimensional and 3D transthoracic echocardiographic studies, using the same ultrasound equipment.
Transthoracic real-time 3D echocardiography was performed using the iE33 system equipped with an X3-1 probe (Philips Medical Systems). Image acquisition was performed from the apical window with the patient in the lateral decubitus position. Full-volume acquisitions were performed in the wide-angle mode, in which electrocardiographically triggered wedge-shaped subvolumes were obtained over 4 to 7 consecutive cardiac cycles during a breath hold (frame rate 16 to 37 frames/s). Care was taken to include the entire left ventricle and the MV apparatus in the pyramidal data set. At the 2 institutions, image acquisition was performed by a single experienced sonographer. The volumetric data sets were digitally stored and then transferred into a personal computer for off-line analysis.
LV endocardial surfaces were obtained semiautomatically from real-time 3D echocardiography using commercial software (4D-LV Analysis; TomTec, Unterschleiβheim, Germany). Briefly, after manual tracing of the endocardial borders on end-diastolic (ED) and end-systolic (ES) frames in the 2-, 3-, and 4-chamber views, the software automatically generated the LV surfaces throughout the cardiac cycle. The operator then manually adjusted the detected surfaces before quantification, if needed. From these surfaces, LV volume–versus–time curves were obtained throughout the cardiac cycle, from which ED and ES volumes were measured and the ejection fraction computed as 100 × (ED volume − ES volume)/ED volume. Additionally, these surfaces were exported and used as the basis for 3D shape analysis.
In addition, ES left atrial areas were obtained from apical 4-chamber 2-dimensional transthoracic echocardiographic images by manually tracing the atrial endocardial contour. All LV and left atrial parameters were normalized by body surface area.
Custom software implemented in the MATLAB environment (The MathWorks, Inc., Natick, Massachusetts) was used to analyze the LV endocardial surfaces. Figure 1 briefly depicts the steps of this analysis, which have described in detail elsewhere: (1) the LV surface was sampled along a helical pattern aligned with the LV long axis from apex (θ = 0) to base (θ = 1), using 64 windings and 36 samples per winding; (2) the obtained signal, s(θ), describing the distance from the long axis to the endocardial surface, was normalized by long-axis length, leading to signal s norm (θ); and (c) global 3D shape indexes were defined by measuring the degree of similarity between s norm (θ), computed from the left ventricle, and the signal s ref (θ) obtained, using the same procedure, from a reference 3D shape built according to geometric properties of the left ventricle (principal moments of inertia; see Maffessanti et al for details). Spherical and conical 3D shape indexes in dimensionless units were computed as shape index = 1 − A/A max , where A is the area between the 2 signals s norm (θ) and s ref (θ), and A max is the total area in the (s,θ) plane, equal to 0.5 by definition.
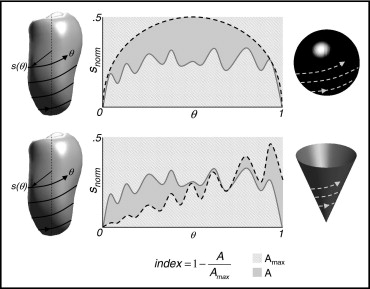
The obtained indexes of sphericity and conicity, computed throughout the cardiac cycle, varied between 0 and 1, with higher values corresponding to better similarity with the relevant reference shape, and were dependent on LV shape but independent of LV dimensions.
To investigate the differences in functional and morphologic parameters due to LV remodeling after MV repair with annuloplasty, analysis of variance for repeated measurements and Tukey’s honestly significantly difference test were performed; p values <0.05 were considered significant.
Moreover, results from the MV group were compared to those obtained from the control group using unpaired Student’s t tests to test the degree of abnormality and its evolution at each time point in the study.
In addition, to test the reproducibility in the computation of the shape indexes, in a subgroup of 25 normal subjects, LV surface detection and the subsequent shape analysis were performed by 2 independent operators, blinded to each other’s measurements. The interoperator variability for LV volume and shape indexes was computed as the coefficient of variation:
Coefficient of variation ( % ) = 1 n ∑ i = 1 n σ μ × 100%,
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree


