The evaluation of aortic root dilation is of major importance for the diagnosis and follow-up of patients with diverse diseases, including the Marfan syndrome. However, we noted that the available nomograms suggested a lower aortic root dilation rate in adults (75%) than in children (90%), when the opposite would have been expected. To establish new nomograms, we selected a population of 353 normal children. We took transthoracic echocardiographic measurements of the aortic root diameters at the level of the aortic annulus, sinuses of Valsalva, sinotubular junction, and ascending aorta according to the American Society of Echocardiography recommendations. All diameters correlated well with the height, weight, body surface area, and age (r = 0.75 to 0.84, p <0.0001). Covariance analysis adjusting for body surface area showed slightly larger diameters at the level of the sinuses of Valsalva in male children than in female children (+1 mm, p = 0.0002). Equations and derived nomograms were developed, giving the upper limit of normal (allowing simple recognition of aortic dilation) and the Z score (allowing fine quantification of dilation and differentiation of normal growth from pathologic dilation) for all 4 aortic root diameters (ie, aortic annulus, sinuses of Valsalva, sinotubular junction, and proximal ascending aorta) according to body surface area and gender. We applied the nomograms to 282 children with confirmed Marfan syndrome, of whom 65.2% presented with dilation of the sinuses of Valsalva. In conclusion, we propose equations to calculate the upper limit of normal and Z-score for aortic root diameters measured by 2-dimensional echocardiography, which should be useful tools for the diagnosis and follow-up of aortic root aneurysms in children.
In our Marfan syndrome (MS) and related diseases multidisciplinary outpatient clinic, we have used recommended nomograms for aortic root diameters assessed by 2-dimensional echocardiography. We noted that aortic root dilation was overdiagnosed in children, because its incidence at the level of the sinuses of Valsalva was lower in adults (75%) than in children <18 years old (90%), when the opposite would have been expected. This observation has also been made by others. The recommended nomograms for aortic root diameters in children were derived from a pediatric population of 52 children referred for innocent precordial murmur. We sought (1) to establish new nomograms using a large population of healthy children, for each of the 4 diameters of the aortic root (eg, aortic annulus, sinuses of Valsalva, sinotubular junction, and proximal ascending aorta) measured by 2-dimensional echocardiography according to the American Society of Echocardiography (ASE) recommendations; and (2) to determine the prevalence of aortic dilation at the level of the sinuses of Valsalva in children with MS, as assessed using these new nomograms.
Methods
All children included in the present study had come to our outpatient clinic devoted to MS from 1995 to 2009 and were ≤18 years old at their first examination. All patients and/or their guardians provided informed consent. Two independent groups of children were retrospectively constituted for the purposes of the present study.
The first group was used to determine the normative data. The main reason for consultation was familial screening because one first-degree relative had been diagnosed with MS. Our rule was to ask all first-degree relatives of affected probands to come for MS screening with a complete clinical and paraclinical evaluation (including genetic, ophthalmologic, cutaneous, orthopedic, and cardiologic examinations) on the same day. Only children in whom no anomaly was observed were included in the present study. All children for whom MS was either confirmed or could not be completely ruled out were excluded, as were patients with a bicuspid aortic valve, a history of familial thoracic aortic aneurysm, or significant aortic regurgitation (defined as moderate or severe).
The second group was a population of children with MS. All patients who came to our clinic for consultation from 1995 and 2009 and were ≤18 years old at the examination with a MS diagnosis fulfilling the international criteria were included in the second group. Only data from the first consultation was kept for each patient when they came several times. This population was used to test our nomograms and to determine the proportion of dilated aortic root diameters in children with MS.
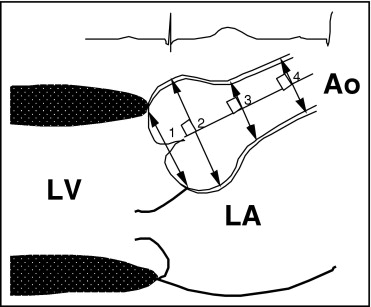
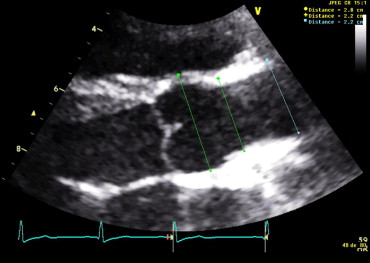
Transthoracic echocardiography was performed by 1 of 5 trained echocardiographists (all cardiologists) using a Sequoia (Siemens Saint-Denis, France) or a Vivid 7 (General Electric, France) ultrasound system. Adequate multifrequency transducers, ranging from 2 to 5 and 3 to 8 MHz, were used. Patients were placed in the left lateral decubitus position, in at rest conditions. The aortic root diameters were measured in 2-dimensional mode at 4 levels (ie, aortic annulus, sinuses of Valsalva, sinotubular junction, and ascending aorta), according to the latest 2005 ASE chamber quantification guidelines. The best parasternal long-axis view was used. Great care was taken to align the echocardiographic plane with the aortic root and to obtain the largest aortic diameters. Appropriately magnified views were used for greater precision. The aortic annulus was measured in systole at the hinge point of the aortic leaflets. The sinuses of Valsalva, sinotubular junction, and proximal ascending aorta were all measured in diastole, perpendicular to the long axis of the aorta, using the leading edge to leading edge technique ( Figures 1 and 2 ). Thus, our measurements included the anterior wall of the aorta and not the posterior wall. The measurements were done the day of the consultation by the cardiologist who did the ultrasound examination, both on-line and off-line (digitized images). The diameters were given in millimeters. The largest of several measurements at each of the 4 predefined levels was kept in the database.
The quantitative data are reported as the mean ± SD. Differences in proportions were tested using the chi-square test.
The body surface area (BSA) was computed using the Dubois and Dubois formula : BSA (m 2 ) = 0.007184 × height (cm) 0.725 × weight (kg) .0.425 The body mass index (BMI) was determined using the Quetelet index: BMI (kg/m 2 ) = weight (kg)/height (m 2 ).
The gender differences for morphologic parameters, age, and aortic root diameters were evaluated using the independent samples t test. The influence of gender on each of the 4 aortic root diameters was further assessed using analysis of covariance to adjust for BSA.
For homogeneity of variance, the values of all 4 aortic root diameters, morphologic parameters (height, weight, BSA, body mass index), and age were transformed to their natural logarithm. Linear regression statistics were then applied to determine the correlations among the logarithmic transformed values of the 4 aortic root diameters, morphologic parameters (height, weight, BSA and its square root, and body mass index), and age. The strength of each correlation was evaluated by Pearson’s correlation coefficient (r). The relation among the logarithmic transformed values of the 4 aortic root diameters, morphologic parameters, and age was described using equations of the form: ln(y) = a.ln(x) + b , with “y” the mean predicted diameter, “a” the regression line multiplier, “x” the morphologic parameter value, and “b” the regression line intercept.
A first set of nomograms displaying the upper limit of normal for the aortic annulus, sinuses of Valsalva, sinotubular junction, and proximal ascending aorta was derived from linear regression analysis of the transformed logarithmic values. The morphologic parameter that correlated best with the aortic diameters was chosen. The upper limit of normal was defined as 2 SDs greater than the mean predicted diameter. The form of the equation giving the upper limit of normal was therefore: ln ( ULN ) = a . ln(x) + b + 2SD, ULN = e (b + 2SD) .(x) a , with ULN, the upper limit of normal, “a” the regression line multiplier, “x” the morphologic parameter value, “b” the regression line intercept, and SD, the standard deviation.
Second, the Z-score equations were established for all 4 aortic root diameters using linear regression analysis of the transformed logarithmic values, with Z defined as the number of SDs greater than or less than the predicted mean diameter: Z = [ln(measured diameter) −ln(predicted mean diameter)]/(SD) , Z = [ln(measured diameter) − (a.ln(x) + b)]/(SD) , with “a” the regression line multiplier, “x” the morphologic parameter value, and “b” the regression line intercept. Of the normal subjects, 95.4% had a Z score of −2% to +2% and 2.3% a Z score of ≥2. Therefore, an aortic diameter can be considered dilated when the Z score is ≥2, with a specificity of 97.7%. Nomograms giving the Z score for the 4 aortic root diameters were derived from these equations.
Results
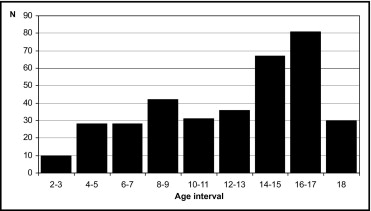
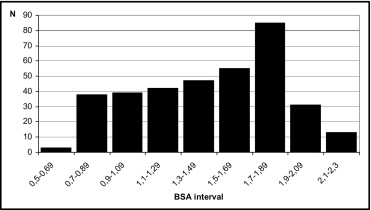
Our first group included 353 children, aged 2 to 18 years old. The male/female ratio was 1.2 (194 male and 159 female children). As shown in Figures 3 and 4 , all age and BSA intervals were well represented, except for the age group of 2 to 3 years old (n = 10) and BSA group of 0.5 to 0.69 m 2 (n = 3). The mean value ± SD of the morphologic parameters, age, and the 4 aortic root diameters are listed in Table 1 . The mean values of age, height, weight, body mass index, and BSA were significantly lower in female children than in the male children (p <0.0001). The mean values of the 4 aortic root diameters were greater in the male children than in the female children (p <0.0001). The difference was 3.1 mm (p <0.0001) at the level of the sinuses of Valsalva. The analysis of covariance indicated that the gender difference remained statistically significant after adjusting for the BSA at the level of aortic annulus and the level of the sinuses of Valsalva. The male children had larger adjusted mean values than the female children: 0.6 mm at the level of the aortic annulus (p = 0.004) and 1 mm at the level of the sinuses of Valsalva (p = 0.0002). In contrast, the adjusted mean of the aortic diameter at the level of the sinotubular junction and ascending aorta was not significantly different.
| Variable | Total Population | Male Children | Female Children | p Value ( t Test) |
|---|---|---|---|---|
| Subjects (n) | 353 | 194 (54.9%) | 159 (45.1%) | |
| Age (years) | 12.7 ± 4.5 | 13.5 ± 4.3 | 11.7 ± 4.4 | <0.0001 |
| Height (cm) | 156.7 (±25.4) | 163.1 (±25.7) | 148.9 (±22.9) | <0.0001 |
| Weight (kg) | 45.5 (±18.4) | 49.9 (±18.7) | 40.2 (±16.6) | <0.0001 |
| Body mass index (kg/m 2 ) | 17.6 (±3.2) | 17.9 (±3) | 17.2 (±3.3) | 0.016 |
| Body surface area (m 2 ) | 1.41 (±0.4) | 1.51 (±0.4) | 1.29 (±0.36) | <0.0001 |
| Aortic annulus (mm) | 18.5 (±3.2) | 19.4 (±3.2) | 17.4 (±2.9) | <0.0001 |
| Sinuses of Valsalva (mm) | 25.9 (±4.6) | 27.3 (±4.7) | 24.2 (±3.8) | <0.0001 |
| Sinotubular junction (mm) | 21.5 (±3.7) | 22.4 (±3.8) | 20.4 (±3.2) | <0.0001 |
| Ascending aorta (mm) | 21.6 (±3.8) | 22.4 (±3.8) | 20.6 (±3.5) | <0.0001 |
Strong correlations were observed between each of the 4 aortic root diameters and age, height, weight, BSA, BSA square root (r = 0.76 to r = 0.85). All were highly significant (p <0.0001). The BMI correlated poorly with all diameters (r = 0.49 to r = 0.54). When all the variables were log transformed, slightly stronger correlations were obtained. The greatest correlation coefficients were found between the log-transformed aortic diameters and log-transformed values of BSA (r = 0.81 to r = 0.85). After dividing the population between the male and female children, the r values were not significantly modified.
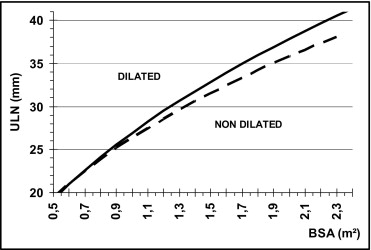
Nomograms displaying the upper limit of normal for the aortic diameter (in millimeters) at the level of the sinuses of Valsalva as a function of BSA (square meters) in the male and female children are presented in Figure 5 . The equations used to build these nomograms are listed in Table 2 , along with those giving the upper limit of normal as a function of BSA and gender for the aortic diameters at the level of the aortic annulus, sinotubular junction, and proximal ascending aorta. These nomograms have been designed to allow easy recognition of aortic dilation in children.
| Aortic Root Level | Gender | ULN Equation | Z Score Equations |
|---|---|---|---|
| Aortic annulus | Male | y = 19.57x (BSA) 0.47 | Z = [ln(d) − 2.78–0.47 × ln(BSA)]/0.1 |
| Female | y = 19.11x (BSA) 0.44 | Z = [ln(d) − 2.75–0.44 × ln(BSA)]/0.1 | |
| Sinuses of Valsalva | Male | y = 26.95x (BSA) 0.49 | Z = [ln(d) − 3.1–0.49 × ln(BSA)]/0.1 |
| Female | y = 26.36x (BSA) 0.44 | Z = [ln(d) − 3.1–0.44 × ln(BSA)]/0.09 | |
| Sinotubular junction | Male | y = 22.29x (BSA) 0.47 | Z = [ln(d) − 2.9–0.47 × ln(BSA)]/0.1 |
| Female | y = 21.76x (BSA) 0.42 | Z = [ln(d) − 2.9–0.42 × ln(BSA)]/0.09 | |
| Proximal ascending aorta | Male | y = 22.74x (BSA) 0.46 | Z = [ln(d) − 2.9–0.46 × ln(BSA)]/0.11 |
| Female | y = 22.20x (BSA) 0.46 | Z = [ln(d) − 2.9–0.46 × ln(BSA)]/0.1 |
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree


