Chapter 5 Gas Exchange
The Basis of Gas Exchange: Ventilation, Diffusion, and Perfusion
Challenges to Lung Function Caused by its Structure
Inequality of ventilation and blood flow: Because the lungs are ventilated through a single main airway (trachea), yet air must reach all 300 million alveoli, there must be a substantial branching airway system. Indeed, some 23 orders of largely dichotomous branching are recognized, resulting in a very large number of very small airways arranged in parallel with each other—much like tree branches emanating and serially dividing from a single trunk. It is impossible to imagine that inhaled air can be distributed homogeneously to all 300 million alveoli, and nonuniform ventilation distribution is well known to occur. Similarly, blood flow reaches the lungs from the main pulmonary artery by a corresponding branching system, and it also is known that perfusion is nonuniform. Nonuniform distribution of ventilation and blood flow are important for gas exchange efficiency as will be shown later.
Wasted ventilation (dead space): The first 17 or so generations of the airways are conducting airways—plumbing whose walls are unable to perform any gas exchange. Their total volume is about 150 mL. This means that with every single breath, 150 mL of inhaled air never reaches the alveoli yet must be moved by muscle contraction. Normally, each breath is about 500 mL in total volume, so about 30% of each breath represents wasted effort. This is not important in health, but in some lung diseases, the effort of breathing is so high that this wasted ventilation, called dead space, leads to insufficient ventilation of fresh gas to the alveoli.
Alveolar collapse: A very large number of very small collapsible structures is potentially physically unstable, due to surface tension forces. The laws of physics show that the pressure inside a soap bubble caused by surface tension is inversely proportional to the bubble radius. To the extent that the soap bubble analogy applies to the alveoli, which simply are not all exactly equal in size, surface tension forces will therefore tend to empty small alveoli into larger alveoli. Unchecked, this progression would lead to massive alveolar collapse with loss of gas exchange surface area and could prove fatal. In fact, the neonatal respiratory distress syndrome is considered to represent an example of just this phenomenon. The body has solved this problem by generating, in normal full-term newborns, a surfactant that lines each alveolus. It reduces surface tension by about an order of magnitude, greatly mitigating the risk of alveolar collapse. What also helps prevent collapse is the aforementioned interdependence whereby adjacent alveoli share common alveolar walls, creating a mesh or network that is inherently self-stabilizing.
Particle deposition: An array of about 20 orders of dichotomous branching leads to a very large (220 in this case) number of small peripheral airways. Although individually each is very small, there are so many of them that their total cross-sectional area becomes very large. With this arrangement, the forward velocity of the air in each small airway is reduced as air is inhaled, which in turn increases the chance that an inhaled dust (or other) particle will settle out and deposit on the small airway wall (compared with larger, more proximal airways, in which the velocity of air flow is much greater). If such a particle is physically, chemically, or biologically dangerous, disease may result, often starting in those small peripheral airways—as is the case for emphysema caused by inhalation of tobacco smoke.
Airway obstruction by mucus: Although the airways have developed a sophisticated particle clearance mechanism using mucociliary transport, the mucus that traps the particles may itself occlude small airways, impairing distal ventilation of the alveoli.
Capillary stress failure: The pulmonary microcirculation is at risk from the inherent structure of the lungs. With capillaries poorly supported in very thin alveolar walls (good for diffusion), they risk rupture into the alveolar space when intravascular pressures rise even modestly. Such alveolar hemorrhage occurs in several conditions, and especially in racehorses, whose lungs are relatively small, leading to high vascular pressures, which in this setting can be fatal.
Pulmonary hypertension: Because all of the cardiac output has to pass through the lungs (compare the systemic circulation, for which flow is divided among all of the body’s other tissues and organs), the potential for high vascular pressures is considerable. The twin processes of capillary distention and recruitment mitigate increases in pressure when perfusion is increased, as in exercise.
Gas Exchange in the Homogeneous Lung: Ventilation, Diffusion, and Perfusion
Ventilation
where 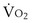 is the volume of O2 taken up into the blood per minute, and
is the volume of O2 taken up into the blood per minute, and  is the minute ventilation, both expressed in L/minute. FIO2 and FEO2 are, respectively, the inhaled and exhaled mean O2 fractional concentrations.
is the minute ventilation, both expressed in L/minute. FIO2 and FEO2 are, respectively, the inhaled and exhaled mean O2 fractional concentrations. 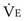 commonly is about 7 L/minute. Because about 21 of every 100 molecules in air are O2 molecules (the rest being mostly nitrogen), FIO2 is 0.21. FEO2 at rest is about 0.17; this difference shows that
commonly is about 7 L/minute. Because about 21 of every 100 molecules in air are O2 molecules (the rest being mostly nitrogen), FIO2 is 0.21. FEO2 at rest is about 0.17; this difference shows that  is about 0.3 L/minute. Because the conducting airways that feed the alveoli do not exchange O2 or CO2, it has become conventional to subtract the volume of gas left in the conducting airways each breath—the so-called anatomic dead space—from the total breath volume before multiplying by respiratory frequency to calculate ventilation, resulting in a variable known as alveolar ventilation (
is about 0.3 L/minute. Because the conducting airways that feed the alveoli do not exchange O2 or CO2, it has become conventional to subtract the volume of gas left in the conducting airways each breath—the so-called anatomic dead space—from the total breath volume before multiplying by respiratory frequency to calculate ventilation, resulting in a variable known as alveolar ventilation (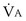 ). Equation 1 then becomes
). Equation 1 then becomes
The tendency is to use partial pressure (PIO2, inhaled; PAO2, alveolar) rather than fractional concentration (FIO2, FAO2) in describing these relationships: From Dalton’s law of partial pressure, PO2 = FO2 × (barometric pressure − water vapor pressure). Allowing for proper units, Equation 2 can then be rewritten as
 is now expressed in mL/minute,
is now expressed in mL/minute, 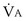 in L/minute, and P in mm Hg.
in L/minute, and P in mm Hg.
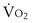 is the whole-body metabolic rate and as such is dictated by the body tissues, not the lungs. Because PIO2 is a constant, Equation 2 can be used to demonstrate the dependence of alveolar PO2 on alveolar ventilation for a given value of
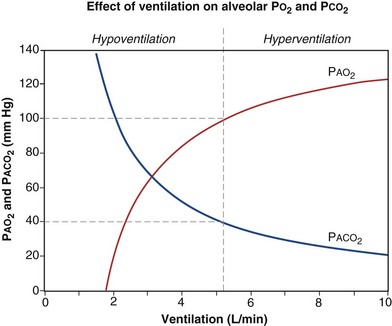
is the whole-body metabolic rate and as such is dictated by the body tissues, not the lungs. Because PIO2 is a constant, Equation 2 can be used to demonstrate the dependence of alveolar PO2 on alveolar ventilation for a given value of 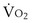 (Figure 5-1). The same concepts apply to CO2, for which it is simpler, because CO2 is essentially absent from inhaled air. The corresponding equation is
(Figure 5-1). The same concepts apply to CO2, for which it is simpler, because CO2 is essentially absent from inhaled air. The corresponding equation is
How ventilation affects PACO2 also is shown in Figure 5-1. It is evident that a relatively small reduction in ventilation will reduce PAO2 and increase PACO2—both substantially.
Dividing Equation 4 by Equation 3 gives
which can be rearranged into what is called the alveolar gas equation:
Diffusion
The laws of diffusion dictate that the rate at which a gas diffuses between two points is the product of the diffusion coefficient for the gas and the partial pressure difference between the two points. In the lungs, the diffusion coefficient, measured as the diffusing capacity, is determined by surface area and distance of the diffusion pathway (see earlier). When a red cell leaves the pulmonary arteries and enters the pulmonary capillary, it arrives with a reduced level of O2, because the tissues visited by that red cell took O2 from the red cell for the tissue’s metabolic needs. The PO2 in the red cell in this blood commonly is about 40 mm Hg. Alveolar PO2, on the other hand, usually is about 100 mm Hg. The large PO2 difference (“driving gradient”) of 60 mm Hg leads to rapid diffusion of O2 from the alveolar gas into the capillary blood. Consequently, however, the blood PO2 increases, reducing the driving gradient, and O2 diffusion slows down as the red cell progresses along the lung capillary network. With modeling of this process, again using mass conservation principles, PO2 is seen to rise approximately exponentially as the red cell moves along the lung capillary until PO2 in the red cell has reached the alveolar value, indicating that diffusion equilibration has occurred. This process is shown in Figure 5-2. Note that for O2, equilibration occurred in about 0.25 second. On average, each red cell takes about 0.75 second to move through the alveolar capillary system, so that diffusion equilibration is complete already a third of the way along the capillary, and thus well before its end. As might be expected, during exercise, time available for a red cell to pick up O2 in the lung is reduced, because blood flow rate is increased, and at very high exercise intensity, there may not be sufficient time for PO2 in the red cell to reach the alveolar value. Accordingly, PO2 in the systemic arterial blood will be lower than that in the alveolus—a situation referred to as hypoxemia caused by diffusion limitation. This effect is seen commonly in exceptional athletes exercising heavily at sea level, and in all subjects exercising at altitude.
While CO2 moves from blood to gas, the principle is the same as for O2. Here, the red cell enters the alveolar capillary with a high PCO2 (because of addition of waste CO2 from tissues visited by the red cell), whereas alveolar PCO2 is lower. Thus, diffusion will move CO2 from red cell to alveolar gas, and red cell PCO2 will fall toward the alveolar value in mirror image to the rise in PO2 described earlier (see Figure 5-2). The speed of equilibration for CO2 is about twice that for O2, so it takes about half the time to reach equilibration. In practice, CO2 is never diffusion-limited. Gases carried in blood only in physical solution (i.e., inert and anesthetic gases) equilibrate even faster—about 10 times as quickly as for O2 (see Figure 5-2). This rule holds true for gases of any solubility.
Gas Exchange
Focusing on O2, Equations 3 and 8 should now be considered together. They both embody mass conservation but express it differently, with Equation 3 reflecting alveolar loss of O2 into blood and Equation 8, red cell gain of O2 into blood. Figure 5-3 shows how, for given constant values of 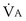 and
and 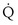 , and for designated values of inspired PO2 (PIO2) and inflowing pulmonary arterial O2 concentration (CvO2),
, and for designated values of inspired PO2 (PIO2) and inflowing pulmonary arterial O2 concentration (CvO2),  would have to vary with alveolar PO2 (to satisfy both these equations) when determined by each of the two equations independently. Because each molecule of O2 that leaves the alveolus by crossing the blood gas barrier appears in the capillary blood, the
would have to vary with alveolar PO2 (to satisfy both these equations) when determined by each of the two equations independently. Because each molecule of O2 that leaves the alveolus by crossing the blood gas barrier appears in the capillary blood, the 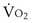 calculated from the two equations must be the same—again, conservation of mass. Thus, only a single value of PAO2 can exist—that at the point of intersection of the two relationships in Figure 5-3. If the calculations in Figure 5-3 were repeated for different values of
calculated from the two equations must be the same—again, conservation of mass. Thus, only a single value of PAO2 can exist—that at the point of intersection of the two relationships in Figure 5-3. If the calculations in Figure 5-3 were repeated for different values of 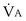 , and thus
, and thus 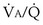 (in this example, keeping
(in this example, keeping 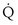 the same), the lines and their point of intersection would change as in Figure 5-4. This figure shows that alveolar PO2 (x axis) and the amount of O2 that can be taken up (
the same), the lines and their point of intersection would change as in Figure 5-4. This figure shows that alveolar PO2 (x axis) and the amount of O2 that can be taken up (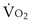 , y axis) both depend on
, y axis) both depend on 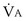 and
and 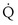 . Commonly, Equations 3 and 8 are combined, because
. Commonly, Equations 3 and 8 are combined, because 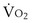 must be the same when calculated from either equation. This yields the ventilation-perfusion equation:
must be the same when calculated from either equation. This yields the ventilation-perfusion equation:
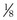 m2 (640-fold less). Moreover, if the same mass of 0.5-µm-thick alveolar wall tissue covering all 300 million alveoli were spread around this one sphere, its thickness would be over 300 µm, also about 600 times greater than in the actual lung. Because diffusion rates depend on the ratio of area to thickness, the real lung is about 640 × 600, or 400,000 times better at diffusive transport than would be a single sphere of the same volume and mass. The message is that by dividing up the lung into a very large number of very small structures, diffusion becomes a feasible and energy-efficient method of gas exchange, circumventing the need for active transport.
m2 (640-fold less). Moreover, if the same mass of 0.5-µm-thick alveolar wall tissue covering all 300 million alveoli were spread around this one sphere, its thickness would be over 300 µm, also about 600 times greater than in the actual lung. Because diffusion rates depend on the ratio of area to thickness, the real lung is about 640 × 600, or 400,000 times better at diffusive transport than would be a single sphere of the same volume and mass. The message is that by dividing up the lung into a very large number of very small structures, diffusion becomes a feasible and energy-efficient method of gas exchange, circumventing the need for active transport.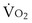 ) can be expressed as the difference between how much O2 was inhaled and how much was exhaled (over that minute). This relation holds because inhaled O2 has only two fates—diffusing into the blood or being exhaled. The amount inhaled is the product of minute ventilation and the concentration of O2 in inhaled air; the amount exhaled is the product of minute ventilation and the concentration of O2 in the exhaled gas. Because O2 concentration is constantly changing during the course of an exhalation, it is appropriate to use the mean concentration over exhalation. Minute ventilation is the product of the volume of each breath (L/breath) and the frequency of breathing (breaths/minute). Although the volumes inhaled and exhaled might be expected to be the same (or the lungs would either blow up or collapse), exhaled volume usually is 1% less than that inhaled because the amount of O2 absorbed into the blood is a little more than the amount of CO2 eliminated from the blood. This small difference can be neglected in most circumstances, as is the case in the following discussion. The mass conservation equation that then describes O2 uptake as a function of ventilation is
) can be expressed as the difference between how much O2 was inhaled and how much was exhaled (over that minute). This relation holds because inhaled O2 has only two fates—diffusing into the blood or being exhaled. The amount inhaled is the product of minute ventilation and the concentration of O2 in inhaled air; the amount exhaled is the product of minute ventilation and the concentration of O2 in the exhaled gas. Because O2 concentration is constantly changing during the course of an exhalation, it is appropriate to use the mean concentration over exhalation. Minute ventilation is the product of the volume of each breath (L/breath) and the frequency of breathing (breaths/minute). Although the volumes inhaled and exhaled might be expected to be the same (or the lungs would either blow up or collapse), exhaled volume usually is 1% less than that inhaled because the amount of O2 absorbed into the blood is a little more than the amount of CO2 eliminated from the blood. This small difference can be neglected in most circumstances, as is the case in the following discussion. The mass conservation equation that then describes O2 uptake as a function of ventilation is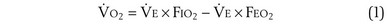
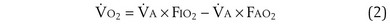
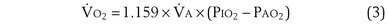
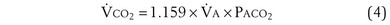
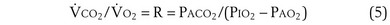
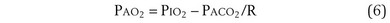

 ) is the amount of O2 in the blood leaving the lungs each minute heading for the left atrium, minus the amount that had entered the lungs in the pulmonary arterial blood. These amounts are the product of the concentration of O2 in each site and the blood flow rate. If Cec
) is the amount of O2 in the blood leaving the lungs each minute heading for the left atrium, minus the amount that had entered the lungs in the pulmonary arterial blood. These amounts are the product of the concentration of O2 in each site and the blood flow rate. If Cec is the blood flow rate through the lungs, mass conservation gives
is the blood flow rate through the lungs, mass conservation gives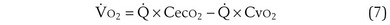
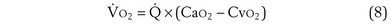

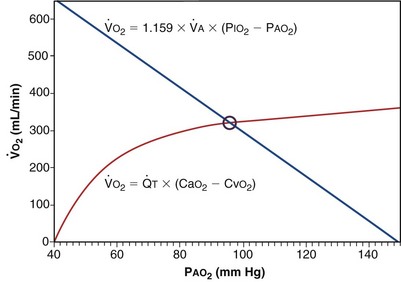
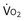 ): one as a function of ventilation (
): one as a function of ventilation (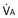 , straight line) and the other based on blood flow (
, straight line) and the other based on blood flow (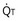 , curved line
, curved line


