Acid–Base Balance and Blood Gas Analysis


Homeostatic system

Action time

Blood buffer systems

Immediate (fraction of second)

Lung (ventilation regulation)

1–15 min

Kidney (alkaline or acid urine elimination)

Hours–days



Blood buffer systems have an immediate action time. They are present in a total amount lower than produced acids (2,400 mEq/L). This difference entails the need for a continuous renovation of buffer systems.

The most important blood buffer is the HCO3 −/H2CO3 system (see Chap.​ 13). Its main role is imputable to three factors: it represents the 65 % of whole buffer power of our body, it has an ubiquitarious distribution (interstitial space (ISS), intracellular space (ICS), plasma, red cells, bones), and it has a pathway of elimination and a pathway of renovation (Rose 1995).

Other buffer systems are hemoglobin, plasma proteins, and phosphate systems.

Hemoglobin lies the H+ (carbaminohemoglobin) formed by the diffusion of CO2 from plasma into erythrocytes (formation and dissociation of H2CO3 for combination with H2O), while bicarbonate returns in the plasma (Rose 1995).

Other plasma proteins present as anion may buffer H+ excess, exchanging Ca2+ with H+: the aminic group NH2 − lies H+, becoming NH3 and releasing Ca2+. (acidosis increases free Ca2+) (Rose 1995). Phosphate ions act as a buffer system in a similar way as bicarbonate, but they are present in very low concentration in the extracellular space (ECS) with respect to bicarbonate, while are very important in maintaining ICS pH (Rose 1995):


$$ {H}_2P{O_4}^{-}\leftrightarrow {H}^{+}+ HP{O_4}^{2-}\leftrightarrow {N}_2 HP{O}_4. $$

The kidneys and lungs eliminate acids or bases in excess, permitting a regeneration of blood buffer systems. When the primitive cause of an acid–base alteration is respiratory, a metabolic compensation develops (see Chap. 14): the kidney eliminates excess H+ and nonvolatile acids, regenerating bicarbonate through their production (from amino acid metabolism) and their increased reabsorption from proximal tubules (acid urine excretion) or increasing bicarbonate elimination and H+ reabsorption (alkaline urine excretion). When the primitive alteration is metabolic, a respiratory compensation develops hyperventilation leads to CO2 elimination when metabolic acidosis occurs, while hypoventilation generates CO2 retention when metabolic alkalosis develops. The lung acts in some minutes, while the kidney in some hours (8–12 h) (Agro and Vennari 2013; Rose 1995).

There are three approaches to interpreting acid–base balance physiology. They use distinct variables derived from a set of master equations that can be transferred from one approach to the other two (Agro and Vennari 2013; Kellum 2000) (Fig. 14.1).

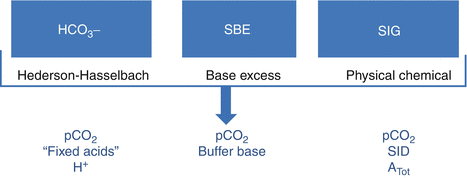
A305677_1_En_14_Fig1_HTML.gif


Fig. 14.1
The three possible approaches to acid–base balance system description. Some factors (i.e., pCO2) are considered by all the approach (Modified from Agro and Vennari (2013, pp. 1–26))



14.2 The Descriptive Approach


The traditional descriptive approach is based on arterial pH, pCO2, and bicarbonate measurements. This approach originated at the end of the nineteenth century, when Henderson revisited the Law of Mass Action from an acid–base equilibrium perspective (Agro and Vennari 2013; Kellum 2005a). The result was


$$ \left[{H}^{+}\right]= Ka\bullet \left[ HA\right]/\left[{A}^{-}\right]. $$
where [H+] is the hydrogen ion concentration in solution, HA is a weak acid, A− a strong base, and Ka is the dissociation constant of the acid. Henderson’s equation revealed that when [HA] = [A−], [H+] does not change as a result of small variations in the amount of acid or base in the solution (Agro and Vennari 2013).

For the H2CO3/HCO3 − system, the relation is


$$ \left[{H}^{+}\right]= Ka\bullet \left[{H}_2C{O}_3\right]/ HC{O_3}^{-}. $$

Considering that H2CO3 is CO2 dissolved in water, the relation may be rewritten:


$$ \left[{H}^{+}\right]= Ka\bullet \left[ pC{O}_2\right]/ HC{O_3}^{-}. $$

In 1917, K.A. Hasselbalch applied Henderson’s equation to the main physiological buffer system (CO2/HCO3 −) using logarithms, giving rise to the Henderson–Hasselbalch equation (Agro and Vennari 2013; Kellum 2005b):


$$ pH= pKa+ \log\;\left(\left[ HC{O_3}^{-}\right]/\left[ pC{O}_2\right]\right) $$

The pCO2 value describes the respiratory contribution (CO2 elimination/retention) to acid–base imbalances, while the metabolic contribution (acid overproduction, accumulation, reduced metabolism) is described by the bicarbonate concentration in the blood. When pCO2 is increased, H+ production increases and a respiratory acidosis develops, while when pCO2 is reduced H+ production reduces, leading to a respiratory alkalosis. When bicarbonate is reduced, free H+ increases and a metabolic acidosis develops, while when bicarbonate increases, free H+ is reduced, causing metabolic alkalosis (see Chap.​ 13).

Since the 1940s, researchers have recognized the limitations of this approach to acid–base physiology: blood bicarbonate concentration is useful in determining the type of acid–base abnormality, but it is not able to quantify the amount of acid or base excess–deficit in the plasma, unless pCO2 is held constant. This observation promoted more researches, in order to quantify the metabolic component (Agro and Vennari 2013; Kellum 2005a).


14.3 The Semiquantitative Approach


In 1957, K.E. Jörgensen and P. Astrup developed a tool to calculate bicarbonate concentration, in which fully oxygenated whole blood was equilibrated with a pCO2 of 40 mmHg at 37 °C. This measurement was called standard bicarbonate. However, subsequent studies determined the role of the other plasma buffer systems (albumin, hemoglobin, and phosphates), which were not considered using either the bicarbonate concentration or the standard bicarbonate method (Agro and Vennari 2013; Astrup et al. 1960).

In 1948, Singer and Hastings defined the sum of the nonvolatile weak acid as the “buffers” and bicarbonates as the “buffer base” (Agro and Vennari 2013; Siggaard-Andersen 1962). This led to several revisions of the method to calculate changes in the buffer base, including the base excess (BE) methodology (Agro and Vennari 2013; Kellum 2005a, b; Astrup et al. 1960; Siggaard-Andersen 1962; Grogono et al. 1976; Severinghaus 1976).

BE is the quantity of metabolic acidosis or alkalosis, defined as how much base or acid should be added to an in vitro whole blood sample to reach a pH of 7.40, while the pCO2 is maintained at 40 mmHg. The most widely used formula for calculating BE is the equation of Van Slyke (Agro and Vennari 2013; Kellum 2005a; Siggaard-Andersen 1977; Wooten 1999; Brackett et al. 1965):


$$ BE=\left( HC{O_3}^{-}-24.4+\left[2.3\times Hb+7.7\right]\times \left[ pH-7.4\right]\right) \times \left(1-0.023\times Hb\right) $$
where HCO3 and hemoglobin (Hb) are expressed in mmol/L.

Subsequently the standard base excess (SBE) was developed (Siggaard-Andersen and Fogh-Andersen 1995). SBE is the BE corrected for the buffer effect of hemoglobin (assuming a mean extracellular hemoglobin concentration of 50 g/L), and it better quantifies the acid–base status in vivo with respect to BE (Agro and Vennari 2013; Brackett et al. 1965; Prys-Roberts et al. 1966):


$$ SBE=0.93\times \left\{\left[ HC{O_3}^{{}^{-}}\right]+14.84\times \left( pH-7.4\right)-24.4\right\}. $$


14.4 The Quantitative Approach


Another approach to acid–base pathophysiology is the calculation of the anion gap (AG), which is the difference in the main measured plasma anion and cation concentrations (Fanzca 2012; Astrup et al. 1960):


$$ \left[\left(N{a}^{+}+{\mathrm{K}}^{+}\right)-\left(C{l}^{-}+ HC{O_3}^{-}\right)\right]=8-16\kern0.24em mEq/\mathrm{L}. $$

The AG corresponds to the difference between nonmeasured anions and cations:


$$ \left[\left(C{a}^{2+}+M{g}^{2+}\right)-\left(P{O_4}^{3-}+S{O_4}^{2-}+ organic\kern0.24em anions+ proteins\right)\right]. $$

Generally, AG values indicate a variation in the concentration of organic acids (lactic acidosis, ketoacidosis). In fact, when their levels increase, the produced H+ consumes bicarbonate, increasing AG values, while the organic anions maintain electric neutrality of the plasma (Agro and Vennari 2013). A possible limit of the AG is the wide variability in both plasma albumin concentrations and renal function with respect to phosphate storage, especially in critically ill patients (Agro and Vennari 2013; Kellum 2005a).

In the 1980s, P. Stewart introduced a new approach finalized to identify dependent and independent variables enrolled in H+ concentration determination (pH) (Agro and Vennari 2013). This approach was based on (Agro and Vennari 2013; Kellum 2005a):



  • The Law of Mass Conservation


  • The electric neutrality


  • Water dissociation constant

Stewart’s approach considers three independent variables (Agro and Vennari 2013; Kellum 2005a):



  • The strong ion difference (SID)


  • Total weak acid concentration (Atot)


  • pCO2

The relationship between SID, pCO2, and Atot is the only determinant of pH, as well as of [HCO3 −], which are dependent variables (Morgan 2005).

SID is the difference in the total amount of strong (totally dissociated) anions and cations:

SID = ([Na+] + [K+] + [Ca2 +] + [Mg2 +])–([Cl−] + [A−] + [SO4 2 –]) (Fig. 14.2).

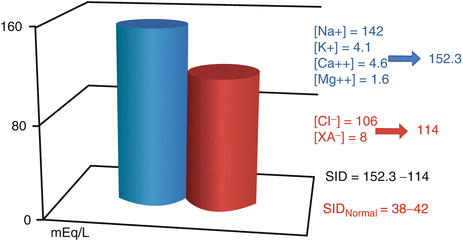
A305677_1_En_14_Fig2_HTML.gif


Fig. 14.2
SID representation. SID strong ion difference, XA − dissociated organic acids (Modified from Agro and Vennari (2013, pp. 1–26))

Considering water electric neutrality and water constant dissociation, it is possible to demonstrate two fundamental principles (Mercieri and Mercieri 2006):

1.

SID is the independent variable influencing H+ and OH− concentrations.

 

2.

SID may vary only adding or reducing strong ions.

 

As a consequence, H+ or OH− variations are index of a primary SID modification, and the primum movens of acid–base alteration is the variation of strong ions concentration. If strong anions increase with respect to strong cations, SID will be negative and H+ are increased with respect to OH−: H+ concentration is the same of the opposite of SID value (electro neutrality principle); while OH− are inversely related to the opposite of SID value (Mercieri and Mercieri 2006):


$$ \left[{H}^{+}\right]=-\left[ SID\right] $$



$$ \left[O{H}^{-}\right]=-{\mathrm{K}}^{\prime}\mathrm{w}/\left[ SID\right]. $$

On the other hand, if strong cations prevail on strong anions, SID is positive. In this case OH− are higher than H+ and OH− are the same of SID value (electro neutrality principle), while H+ are inversely related to SID (Mercieri and Mercieri 2006):


$$ \left[O{H}^{-}\right]=\left[ SID\right] $$



$$ \left[O{H}^{-}\right]=-{\mathrm{K}}^{\prime}\mathrm{w}/\left[ SID\right]. $$

SID is physiologically positive (38–42 mEq/L). To preserve electric neutrality it must be balanced by a corresponding excess of negative charges, represented by a dissociated weak acid such as HCO3 −, proteins (especially albumin), phosphate, and minimal concentrations of CO3 2− and OH−. Generally, proteins, phosphate, and other nonvolatile acids are indicated as A− (Kellum 2005a; Mercieri and Mercieri 2006; Kellum et al. 1995).

As a consequence (Mercieri and Mercieri 2006):


$$ SID= bicarbonate+{\mathrm{A}}^{-}. $$

The SID value obtained with this formula is called effective SID (eSID) vs the apparent SID (aSID), obtained by the calculus of the sum of strong anions and strong cations. When there is a difference between aSID and eSID, a strong, nonmeasured anion is present (i.e., lactate, ketoacids), and it is causing a consumption of buffer systems (bicarbonate and A−), with a reduction of eSID (Mercieri and Mercieri 2006).

The balance between aSID and eSID is defined as SID gap (SIG) and is an index for strong anions that are not measurable (XA−) (Fencl et al. 2000):


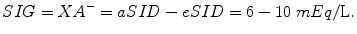
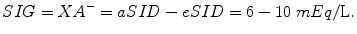
$$ SIG=X{A}^{-}= aSID- eSID=6-10\; mEq/\mathrm{L}. $$

In body fluid compartments there are varying concentrations of nonvolatile weak acids. In plasma they are represented by inorganic phosphate and albumin. The same applies to ISS, although total concentrations here are very small. In red cells the predominant source is hemoglobin (Morgan 2005).

The undissociated form of weak acids (HA) is neutral; the dissociated form (A−) is negative. Their concentrations reflect the Law of Mass Conservation


$$ \left(\left[ Atot\right]=\left[{\mathrm{A}}^{-}\right]+\left[ HA\right]\right) $$
and the dissociation equilibrium


$$ \left(\left[{\mathrm{H}}^{+}\right]*\left[{\mathrm{A}}^{-}\right]= Ka*\left[ HA\right]\right). $$

As a consequence A−, HA, and H+ may vary with pH, but Atot remains the same and is the independent variable influencing other parameters (Mercieri and Mercieri 2006).

When CO2 is present in water solution, it originates four kinds of molecules: dissolved CO2, H2CO3, HCO3 −, and CO3 2−. These species are involved in a chemical reaction in our body resulting in effects on acid–base balance (Mercieri and Mercieri 2006).

Adding CO2 to solutions (body fluids) containing strong ions and weak acids, Stewart needed six equations to describe acid–base balance modification (Table 14.2).

Knowing the independent variables (pCO2, SID, Atot), the system may be solved for the remaining unknown variables ([A−], [HCO3 −], [OH−], [CO3 2−], [HA], and [H+]) (Morgan 2005).


Table 14.2
The six equations of Stewart’s approach




























Physical and chemical law
Equation

Water constant dissociation
[H+] × [OH−] = K′w

Weak acid constant dissociation
[H+] × [A−] = Ka × HA

Mass conservation law
[HA] + [A−] = Atot

Acid carbonic constant dissociation
[H+] × [HCO3 −] = Kc × pCO2

Bicarbonate constant dissociation
[H+] × [CO3 2 −] = Kc × [HCO3 −]

Electric neutrality
SID + [H+]–[H+] − [HCO3 −] − [CO3 2 −] − [A−] − [OH−] = 0

Resolving the system for pH (−log [H+]), a resultant is a simplified equation that may be written as (Mercieri and Mercieri 2006)


$$ pH= pK1+ \log\;\left\{ SID+-\left[ ATOT/\left(1+10{\mathrm{p}}^{Ka- pH}\right)\right]\right\}/ pC{O}_2. $$

When SID = HCO3 − and Atot = 0, the equation becomes the same of Henderson–Hasselbalch (Mercieri and Mercieri 2006):


$$ pH= pK1+ \log\;\left[ HC{O_3}^{-}\right]/ pC{O}_2. $$


14.5 Relation Among Fluid, Electrolyte, and Acid–Base Balance


In the 13th chapter and in the previous paragraphs, it has been evidenced that body homeostasis is mainly based on three principles (Fig. 14.3):

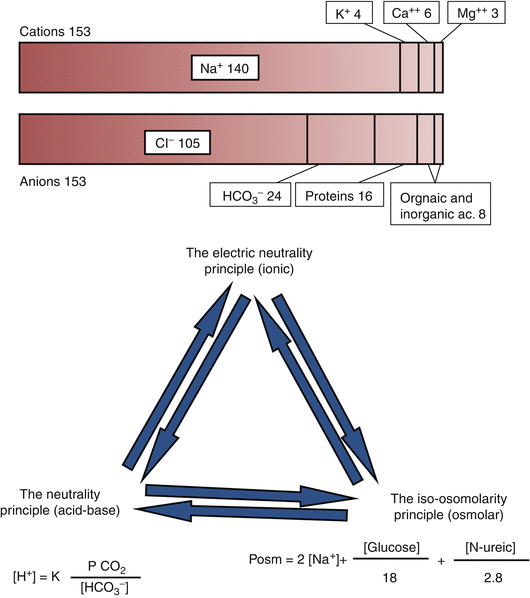
A305677_1_En_14_Fig3_HTML.gif


Fig. 14.3
Relation among the ionic, acid–base, and osmolar balances




  • The electric neutrality principle (ionic)


  • The iso-osmolarity principle (osmolar)


  • The neutrality principle (acid–base)

These three principles are strictly interconnected as is immediately evident from Stewart’s approach to acid–base balance.

Hydro-electrolytic and acid–base balances are related by bicarbonate: it is present in the Gamble gram and in the Henderson–Hasselbalch equation. Variation of bicarbonate plasma levels may affect the electric neutrality and the neutrality principles. Hydrogen ions are also present in Gamble ionogram and in the Henderson–Hasselbalch equation.

Osmolar and hydro-electrolytic balances are mainly connected by sodium, present in Gamble gram and osmolarity equation, where sodium concentration is multiplied for two. As a consequence, little sodium variation may have great influences on osmolarity. Generally sodium is contained in the plasma as salts such as NaHCO3 or NaCl.

If bicarbonates are reduced other anions have to increases in order to maintain electric neutrality. Chloride and bicarbonates are strictly related and their sum must be constant in any moment: if one of them increases, the other reduces and vice versa, in a rate of 1:1. In order to maintain electric neutrality, when both chloride and bicarbonates are reduced, other anions concentration should be increased (see Gamble gram and AG) (Sgambato and Prozzo 2003; Vulterini et al. 1992).

Finally, all acid–base interventions, including fluid administration, act through SID (electrolyte, osmolarity) or Atot or in combination (Morgan 2005; Sgambato and Prozzo 2003).

The clinical consequences of the relations between the three systems are presented in some examples:

1.

In case of diarrhea, bicarbonate will be reduced. To respect electric neutrality principle, a hypobicarbonatemia causes hyperchloremia. It is also responsible of a variation in Henderson–Hasselbalch equation with an increase of hydrogen ion concentration and the development of metabolic acidosis.

 

2.

In case of vomitus, there is a loss of HCl. In order to respect electric neutrality, chloride reduction is replaced by bicarbonate increase. The loss of hydogenions and the reduction of bicarbonate increase will determine a metabolic alkalosis (accompanied by hypochloremia). In case of prolonged vomitus, to restore fluid and chloride deficit fluid are administered. The restoration of chloride levels determines a reduction of bicarbonate and, consequently, a correction of alkalosis.

 

3.

Bicarbonate is present in the plasma as sodium salt. As a consequence, any variation in bicarbonate levels will modify sodium and osmolar balance and vice versa. For example, when bicarbonate (NaHCO3) is administered to correct a severe metabolic acidosis, osmolarity increases potentially developing hypervolemia. Moreover, sodium overload determines a reduction of other cations (K+ or Ca2+).

 


14.6 Basis of Pathophysiology of Acid–Base Balance in the Postoperative ICU Setting of Cardiac Surgery


Maintaining acid–base balance during and after cardiac surgery is essential for the success of the surgery, especially for procedures requiring prolonged bypass time. As an example, cardiac surgery patients are at high risk for developing arrhythmias: the presence of a neutral pH is necessary to obtain a response to pharmacological and electric treatments. At the same time acid–base status is considered as an index of adequate perfusion of tissue (i.e., lactate increase, adequate renal compensation) and may modify blood flux distribution. Moreover pH and pCO2 variation may influence Hb-curve dissociation reducing Hb saturation (acidosis, pCO2 increases) or reducing Hb capacity to transfer O2 to tissue (alkalosis, pCO2 decreases) (Agrò et al. 2013). Literature demonstrated in experimental and clinical studies the influence of pH on vascular tone resulting in possible blood flux redistribution and blood pressure alteration (Celotto et al. 2008). Both modification of ECS pH (pHe) and ICS pH (pHi) may cause these alterations through many proposed mechanisms: neurotransmitter release, prostanoids, purines, smooth cell hyperpolarization, NO, and changes in intracellular calcium concentration (Franco-Cereceda et al. 1993; Aalkjaer and Poston 1996; Ishizaka and Kuo 1996). Moreover, acid–base balance alterations have been related to modification of endothelium activity, with different effects according to the type of considered vessel (Celotto et al. 2008).

On the other hand, cardiac surgery is responsible for profound alteration in acid–base system. The mechanism at the base of this modification is different, causing an impact on acid–base balance in opposite directions (Dobell et al. 1960; Beecher and Murphy 1950; Gibbon et al. 1950; Puyau et al. 1962). Some of these mechanisms depend on the type of oxygenator and the type of blood flow during the CPB and CPB duration, the kind and the duration of postoperative mechanical ventilation, hypotension in the postoperative setting (need for inotropes and/or vasoactive drugs, bleeding), kind of fluid used for priming and for liquid management (balanced vs unbalanced), temperature modifications (hypothermia reduces buffer system dissociation, determining a “natural alkaline shift,” while CO2 becomes more soluble and pCO2 is decreased), and hemolysis (Fanzca 2012; Ito et al. 1957; Coffin and Ankeney 1960; Litwin et al. 1959; Kirklin et al. 1956).

The more frequent alteration of acid–base equilibrium in cardiac patients is metabolic acidosis (Fanzca 2012). It is thought to be due to preexisting respiratory alkalosis, increased lactate levels, hypoxia, and hypoperfusion (Dobell et al. 1960; Beecher and Murphy 1950; Gibbon et al. 1950; Puyau et al. 1962; Ito et al. 1957). Acidosis induces systemic vasodilatation (included coronary) and pulmonary vasoconstriction (Fanzca 2012). Although vasodilatation may have positive effect, such as an increase in coronary blood flow, its consequence may be detrimental in patients presenting a cardiac dysfunction after the surgery (Celotto et al. 2008; Clancy and Gonzalez 1975; Ely et al. 1982). Moreover, acidosis reduces the responsiveness to catecholamines, decreasing pharmacological effectiveness of the treatment of postoperative hemodynamic instability and further precipitating patients’ conditions. Pulmonary vasoconstriction may increase pulmonary resistance and decompensate the hemodynamic and respiratory status of cardiac surgery patients (Celotto et al. 2008).


14.7 Fluid and Electrolyte Management Consequences on Acid–Base Balance


The previous chapter evidenced the relation among fluid administration and modification of acid–base status, according to fluid properties. Moreover, Stewart’s approach underlined the role of electrolyte in maintaining or modifying acid–base balance. As a consequence, the debate on SID of infused solution has adjunct to literature discussion.


14.7.1 Hyperchloremic Acidosis


When establishing fluid therapy, both acid–base and electrolyte iatrogenic disorders must be avoided. Generally, metabolic acidosis with hyperchloremia is the most frequently induced alteration (Agro and Benedetto 2013). Many available solutions do not contain Atot (anions), leading to a dilution of ECS Atot and, consequently, to a metabolic alkalosis. However, this effect is commonly overwhelmed by the increase of the SID with the infusion, contributing to acidosis development (Morgan 2005). Clinical studies have revealed that chloride excess causes a specific splanchnic and renal vasoconstriction, interferes with cellular exchanges, and reduces the glomerular filtration rate (GFR), leading to sodium and water retention (Agro and Benedetto 2013; Quilley et al. 1993; Wilcox 1983). Hyperchloremia is generally associated with metabolic acidosis and may cause a further reduction in GFR (Agro and Benedetto 2013; Wilcox 1983). It has been shown that balanced and plasma-adapted solutions help to avoid hyperchloremic acidosis, while assuring the same volume effect as unbalanced solutions and potentially reducing morbidity and mortality (Agro and Benedetto 2013; Zander 2006).


14.7.2 Dilution Acidosis


Currently available solutions used throughout the world do not contain the physiological buffer base bicarbonate because it cannot be incorporated into polyelectrolyte solutions, since carbonate precipitation would occur (Agro and Benedetto 2013). For this reason, any fluid infusion may cause “dilution” acidosis, i.e., a dilution of the HCO3 – concentration, while the CO2 partial pressure (buffer acid) remains constant (Agro and Benedetto 2013; Shires and Holman 1948; Asano et al. 1966). In the recent literature, the classic view of dilution acidosis has been reviewed. In fact, according to Stewart’s approach, bicarbonate is a dependent variable, while the SID of the infused solution is the determinant of acid–base effects. When SID of used fluid is zero, such as saline solutions with [Cl−] = [Na+], dextrose, mannitol, or water, the administration of large volume leads to a reduction (dilution) of SID, causing metabolic acidosis, independently from plasma [Cl−] variations (Makoff et al. 1970; Miller and Waters 1997; Storey 1999; Figge et al. 1998). Metabolic acidosis may have catastrophic consequences, especially in patients with preexisting acidosis (i.e., patient with reduced CI and hypoperfusion) (Agro and Benedetto 2013).


14.7.3 SID


In order to avoid iatrogenic alteration of acid–base balance, an infused solution should reduce SID (acidifying power) in a minimal rate, necessary to counteract the Atot dilution alkalosis. As a consequence, the concept of balanced solution should be extended considering SID and, particularly, the need for a SID lower than plasma, but higher than zero (Morgan 2005). The ideal SID value is 24 mEq/L. It means that 24 mEq/L of the strong anion Cl− should be replaced by other anions, such as metabolizable anions (Morgan 2005; Morgan et al. 2004).


14.7.4 Metabolizable Anions and Base Excess


To compensate the absence of bicarbonate, initially OH−, HCO3 −, and CO3 2− were adjuncted in vitro as components of IV fluids. It was immediately evident they rapidly equilibrate with CO2 (Morgan 2005). To overcome this problem, metabolizable anions were used. Metabolizable anions are organic anions that may be converted to HCO3 − by tissues. The main metabolizable anions are gluconate, malate, lactate, citrate, and acetate. In IV fluid, the most frequently used metabolizable ions are acetate, malate, and lactate (Agro and Benedetto 2013).

Acetate and malate are contained in plasma in very low concentrations. They may be metabolizable in all tissues, especially in muscles, liver, and heart (Agro and Benedetto 2013; Lundquist 1962). Acetate is an early-onset (within 15 min) alkalinizing anion, while malate has a slower action (Agro and Benedetto 2013; Mudge et al. 1949; Knowles et al. 1974; Akanji et al. 1989).

The most commonly used metabolizable anion is lactate, which is normally produced in the human body. In fact, lactate is the main product of anaerobic glycolysis. It is metabolizable only by the liver (Agro and Benedetto 2013). However, the use of lactate has been the cause of debate in clinical practice and in the literature, especially with respect to patients with preexisting lactic acidosis. This condition is a manifestation of disproportionate tissue lactate formation, with respect to hepatic lactate metabolism (Agro and Benedetto 2013; Johnson et al. 1969; Levraut et al. 1998). Lactate levels are major criteria in the routine evaluation of critically ill patients; indeed, changes in lactate concentration can provide an early and objective evaluation of patient responsiveness to therapy (Agro and Benedetto 2013; Abramson et al. 1993; Bakker et al. 1996; Cowan et al. 1984; Falk et al. 1985; Friedman et al. 1995; Henning et al. 1982; McNelis et al. 2001; Vincent et al. 1983; Rivers et al. 2001). Furthermore, plasma lactate levels in the first 24–48 h have a high predictive power for mortality in patients with various forms of shock, including cardiac, hemorrhagic, and septic shock (Agro and Benedetto 2013). In these situations, the administration of lactate-containing fluids may exacerbate the already existing lactic acidosis and interfere with lactate monitoring for diagnostic purpose (Agro and Benedetto 2013; Levraut et al. 1998; Weil and Afifi 1970). According to these evidences, common sense suggests that in ICU patients any use of lactate-containing solutions should be avoided (Agro and Benedetto 2013).

Another indicator of acidosis is base excess (BE). Since 1990, clinical trials have demonstrated that evaluating BE at the time of admission of critically ill patients is indeed the best prognostic indicator for mortality, complication rate, and transfusion needs (Agro and Benedetto 2013). Persistent base excess disorders above or below 4 mmol/L differ with respect to mortality rates: 9 and 50 %, respectively (Agro and Benedetto 2013; Kincaid et al. 1998).

Balanced, plasma-adapted solutions reduce the risk of acidosis and BE alterations (Agro and Benedetto 2013).


14.7.5 Crystalloids and Acid–Base Status


The composition of widely used crystalloids is discussed in the 13th chapter (see Table 13.​3).

Normal saline solution is a solution with SID = 0, and literature has extensively demonstrated that it causes metabolic acidosis, especially after large amount of infusion during normovolemic hemodilution or for cardiopulmonary bypass (Morgan 2005; Morgan et al. 2004; Beers 2006; Scheingraber et al. 1999; McFarlane and Lee 1994; Prough and Bidani 1999; Rehm et al. 2000; Hayhoe et al. 1999; Liskaser et al. 2000; Himpe et al. 2003). In addition to renal effects (discussed in chapter 14th), metabolic acidosis inhibits cardiac contractility, adrenoceptor function, and coagulation (Fanzca 2012).

Considering a stable and normal plasma lactate concentration of 2 mEq/L, Ringer lactate and Ringer acetate SID is 27 mEq/L. As a consequence, they are slightly alkalinizing solutions, reducing metabolic acidosis risk, typical of first-generation crystalloids (Morgan 2005; Reid et al. 2003; Traverso et al. 1986; Waters et al. 2001).

Both Ringer solutions are more plasma-adapted than normal saline but are nonetheless unbalanced.

Last generation crystalloids are isotonic, balanced, and plasma-adapted solutions that reduce the risk of chloride excess and dilution acidosis, with a decreased influence on lactate monitoring, lactic acidosis, and base excess (BE). They have a SID higher than zero but lower than plasma (SID ~ 29 mEq/L) and, like Ringers solutions, have an alkalinizing power (Morgan 2005).


14.7.6 Colloids and Acid–Base Status


As for crystalloids, SID value of each colloid is an important feature to consider before the administration. The possible effects of their SID is reduced by two factors: the lower amount infused with respect to crystalloids and their possible contribution to Atot (the colloidal molecules may be weak acid) (Finfer et al. 2004; Liskaser and Story 1999). As a consequence, Atot dilution alkalosis is reduced, as long as colloidal molecules persist in the extravascular space (Morgan 2005). However, after the infusion, weak acid colloids with SID > 0, such as albumin (HA) and gelatins, presented a tendency to induce metabolic acidosis similar to 0.9 % saline solution and other colloids with SID = 0 (Morgan 2005).

Currently available HA solutions are prepared as NaCl solutions (SID =0), which can lead to metabolic acidosis and hyperchloremia and interfere with sodium and water excretion, thus impairing renal function, especially in hypovolemic shock patients. In acute renal failure, HA may accumulate after its massive administration (Morgan 2005).

Succinylated gelatins are dispersed in a 4 % polyelectrolyte solution generally containing Na+ 154 mEq/L, K+ 0.4 mEq/L, Ca2+ 0.4 mEq/L, and Cl−120 mEq/L (effective SID = 34). Their low chloride content reduces the risk of hyperchloremic acidosis and may be helpful in patients with acid–base alterations.

Recent balanced HES presented SID closer to the ideal value (24 mmEq/L). They have been demonstrated to reduce the risk of iatrogenic metabolic acidosis and potentially improve gastric mucosal blood flow, with a possible impact on endotoxemia survival (Wilkes et al. 2001; Kellum 2002). In a prospective, randomized, double-blind study of cardiac surgery patients, a balanced HES 130/0.4 preparation was compared to an unbalanced HES 130/0.4: while the hemodynamic status did not differ between the two groups, the base excess was significantly less negative in the balanced than in the unbalanced HES group (Base et al. 2011).


14.8 Maintaining Acid–Base Balance



14.8.1 Arterial Blood Gas Analysis Interpretation


The evaluation of seriated arterial blood gases analysis (ABG) appears as indispensable to guide the postoperative management of the cardiac surgery patients and to permit a precocious diagnosis of tissue hypoperfusion and DO2 modification. In fact, ABG gives direct information about the main determinant of DO2 (pO2, SpO2, and Hb) and indirect information about CI, tissue perfusion, and oxygenation (lactic acidosis, hypoxic acidosis). Moreover it allows the identification of ionic, osmolar, and acid–base alterations. Acid–base alteration are often cause of matter in clinical practice, they should be unrecognized and have catastrophic impact on patients.

According to acid–base perspective, ABG interpretation may use one of the approach previously presented (Henderson-Hasselbalch, BE, AG, or Stewart’s approach) or more of one of them, in order to better understand the contemporary ionic and osmolar alterations and the cause of acid–base disturbance.

Examining ABG it is important to take into account that pH, pCO2, and pO2 are measured while HCO3 − and BE values are calculated.

To evaluate the acid–base state of the patient, the first value to consider is pH.

A pH <7.38 indicates acidemia; a pH >7.42 indicates alkalemia. According to the presence of buffer and compensatory systems, pH may be normal, but the other parameters may be changed indicating alkalosis or acidosis. This situation is the more frequent in the clinical practice and requires a profound knowing of physiology and of the meaning of the different parameters obtainable with ABG.

Only gold members can continue reading. Log In or Register to continue

Related posts:

  1. Cardiovascular Pharmacology
  2. Postoperative Considerations of Cardiopulmonary Bypass in Adult Cardiac Surgery
  3. Risk and Outcome Assessments
  4. Fluid Management and Electrolyte Balance

Stay updated, free articles. Join our Telegram channel
Tags:
Feb 28, 2017 | Posted by in CARDIOLOGY | Comments Off on Acid–Base Balance and Blood Gas Analysis

Full access? Get Clinical Tree
Get Clinical Tree app for offline access